WADE: from Lisbon to the world
The Lisbon Webinar in Analysis in Differential Equations is a joint iniciative of CAMGSD, CMAFcIO and GFM, three research centers of the University of Lisbon. It is aimed at filling the absence of face-to-face seminars and wishes to be a meeting point of mathematicians working in the field.
All seminars will take place in the Zoom platform. The links for the planned seminars can be found here. In order to get the password to access the seminars, please subscribe the announcements in the registration menu, or contact one of the organizers.
Next webinar | https://wade.ulisboa.pt/
29.05.2024 | 14:00 — 15:00 Europe/Lisbon (room P3.10, Mathematics Building, IST - ONLINE)
José Ramón Madrid Padilla (Virginia Tech)
Convolution Inequalities and Applications
In this talk we will discuss a collection of convolution inequalities for real valued functions on the hypercube, motivated by combinatorial applications.
07.03.2024 | 13:30 — 14:30 Europe/Lisbon (room 6.2.33 - Faculty of Sciences, University of Lisbon)
Carlos N. Rautenberg (George Mason University)
Variational Problems on Measure Spaces with Constraints
We consider a class of variational problems involving partial differential operators on non-standard vector-valued measure spaces with divergences (represented by measures or by functions). This class of problems arise from the study of the growth of heterogeneous sand piles and via (Fenchel) duality of the aforementioned models. Further, mixed boundary conditions are in place and these are established by means of a normal trace characterization of the vector measure. We determine existence of solutions, stability and strong duality results. We finalize the talk with numerical tests.
21.02.2024 | 13:10 — 14:10 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Matthias Hofmann (Texas A&M)
Computing eigenvalues of the discrete $p$-Laplacian via graph surgery
We discuss the dependence of the eigenvalues and eigenfunctions for the discrete signed $p$-Laplacian under perturbation by a cut parameter. In particular, we prove a formula for the derivative of the eigenvalues and show that the eigenvalues of the discrete signed $p$-Laplacian on the original graph can be characterized via extremal points of the perturbed system. In this context, we elaborate on how graph surgery can be used in order to compute eigenvalues of the discrete (signed) $p$-Laplacian by looking at some examples. The derivation formula is reminiscent of the formula for linear eigenvalue problems given by the Hellmann-Feynman theorem and our results extend previous results for the linear case $p=2$ attained by [Berkolaiko, Anal. PDE 6 (2013), no. 5, 1213-€“1233].
15.02.2024 | 13:30 — 14:30 Europe/Lisbon - room 6.2.33, Faculty of Sciences of the Universidade de Lisboa
Damião Araújo (Universidade Federal da Paraíba)
Regularity in diffusion models with gradient activation
In this talk, we discuss sharp regularity estimates for solutions of highly degenerate fully nonlinear elliptic equations. These are free boundary models in which a nonlinear diffusion process drives the system only in the region where the gradient surpasses a given threshold. This is joint work with Aelson Sobral, Universidade Federal da Paraíba - Brazil, and Eduardo Teixeira, University of Central Florida - EUA.
08.02.2024 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Yingfei Yi (University of Alberta)
Quasi-Stationary Distributions
Quasi-stationary distributions (QSDs) are those almost invariant to a diffusion process over exponentially long time. Representing important transient stochastic dynamics, they arise frequently in applications especially in chemical reactions and population systems admitting extinction states. This talk will present some rigorous results on the existence, uniqueness, concentration, and convergence of QSDs along with their connections to the spectra of the Fokker-Planck operators.
08.02.2024 | 14:30 — 15:30 Europe/Lisbon (FCUL | room 6.2.33)
Hyeong-Ohk Bae (Ajou University, Republic of Korea)
Interaction of particles and an incompressible fluid
We present a new coupled kinetic-fluid model for the interactions between Cucker-Smale (C-S) flocking particles and incompressible fluid on the periodic spatial domain Td and in an infinite channel.
Our coupled system consists of the kinetic Cucker-Smale equation and the incompressible Navier-Stokes equations, and these two systems are coupled through the drag force. For the proposed model, we provide a global existence of weak solutions and a priori time-asymptotic exponential flocking estimates for any smooth flow, when the kinematic viscosity of the fluid is sufficiently large. The velocity of an individual C-S particle and fluid velocity tend to the averaged time-dependent particle velocities exponentially fast.
25.01.2024 | 14:30 — 15:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Cho Chuhee (Seoul National University, Republic of Korea)
Almost everywhere convergence of sequence of Schrödinger means
In this talk, we consider pointwise convergence of the Schrödinger means along sequences $t_n$ that converge to zero. We discuss sufficient conditions for the convergence and explain the key observation, which is that bounds on the maximal function $\sup_{n} |e^{it_n\Delta} f| $ can be deduced from those on $\sup_{0\lt t\le 1} |e^{it\Delta} f|$ when $\{t_n\}$ is contained in the Lorentz space $\ell^{r,\infty}.$ We will discuss sharp counterexamples for the related maximal estimates.
25.01.2024 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
David Beltran (Universitat de València)
The helical maximal function
Consider the maximal function associated with averages over dilates of the helix (or, more generally, of any curve with non-vanishing curvature and torsion). This object can be seen as a 3-dimensional analogue of the classical circular maximal function in the plane, studied by Bourgain (sharp $L^p$ bounds for $p>2$) and Schlag and Schlag-Sogge (sharp $L^p$-$L^q$ bounds). In this talk, we report sharp 3-dimensional versions of those well-known 2-dimensional results, which use recent developments in multilinear harmonic analysis. This is based on joint works with Shaoming Guo, Jonathan Hickman and Andreas Seeger ($L^p$ bounds), and Jennifer Duncan and Jonathan Hickman ($L^p$-$L^q$ bounds).
24.01.2024 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Seheon Ham
(Seoul National University, Republic of Korea)
Remarks on dimension of union of curves
We study an analogue of Marstrand's circle packing problem for curves in higher dimensions.
We consider collections of curves which are generated by translation and dilation of a curve $\gamma$ in $\mathbb R^d$, i.e., $ x + t \gamma$, $(x,t) \in \mathbb R^d \times (0,\infty)$.
For a Borel set $F \subset \mathbb R^d\times (0,\infty)$, we show the unions of curves $\bigcup_{(x,t) \in F} ( x+t\gamma )$ has Hausdorff dimension at least $\alpha+1$ whenever $F$ has Hausdorff dimension bigger than $\alpha\in (0, d-1)$.
We also obtain results for unions of curves generated by multi-parameter dilation of $\gamma$.
One of the main ingredients is a local smoothing type estimate (for averages over curves) relative to fractal measures.
This talk is bases on recent work with Herym Ko, Sanghyuk Lee, and Sewook Oh.
7.12.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Andreas Seeger (University of Wisconsin-Madison)
The Nevo-Thangavelu spherical maximal function on two step nilpotent Lie groups
Consider $\mathbb R^d\times \mathbb R^m$ with the group structure of a $2$-step Carnot Lie group and natural parabolic dilations. The maximal operator originally introduced by Nevo and Thangavelu in the setting of the Heisenberg groups is generated by (noncommutative) convolution associated with measures on spheres or generalized spheres in $\mathbb R^d$. We discuss a number of approaches that have been taken to prove $L^p$ boundedness and then talk about recent work with Jaehyeon Ryu in which we drop the nondegeneracy condition in the known results on Métivier groups. The new results have the sharp $L^p$ boundedness range for all two step Carnot groups with $d\ge 3$.
28.11.2023 | 13:30 — 14:30 Europe/Lisbon (FCUL | room 6.2.33)
Elvira Zappale (Università di Roma "La Sapienza")
Optimal design problems and applications to thin structures
I will discuss several results, mostly obtained in collaborations with several authors, dealing with the optimal design problems, both in the framework of linear and nonlinear elasticity. Among the several models presented I will focus also on the case in which the hyperelastic energy density has nonstandard, possibly variable, growth. The case of thin structures will also be considered.
16.11.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Mateus Costa de Sousa (Basque Center for Applied Mathematics)
Sharp embeddings between weighted Paley–Wiener spaces
In this talk we will discuss some extremal problems related to embeddings between weighted Paley–Wiener spaces. We will present some asymptotic results for sharp constants in terms of the parameters involved, deduce existence results for extremal functions as well as radial symmetry of those, and talk about some numerical results. For certain cases, these extremal problems can be reformulated in terms of sharp Poincaré inequalities, and for those cases we will present a characterisation of extremizers and sharp constants that recover several classical results.
16.11.2023 | 14:30 — 15:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Héctor Chang Lara, (CIMAT, Mexico)
A transmission problem for first and second order operators
We establish the existence and uniqueness of viscosity solutions within a domain $\Omega\subseteq \mathbb{R}^n$ for a class of equations governed by elliptic and eikonal type equations in disjoint regions. Our primary motivation stems from the Hamilton-Jacobi equation that arises in the context of a stochastic optimal control problem.
27.10.2023 | 11:00 — 12:00 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Christoph Thiele (Universität Bonn)
Quantum signal processing and nonlinear Fourier analysis
Elaborating a connection with nonlinear Fourier analysis, we extend a well known algorithm in quantum signal processing to represent measurable signals by square summable sequences. The coefficients of the sequence depend Lipschitz continuously on the signal in suitable metric.
This is joint work with Michel Alexis and Gevorg Mnatsakanyan.
20.10.2023 | 13:30 — 14:30 Europe/Lisbon (FCUL | room 6.2.33)
Daniele Cassani (Università degli Studi dell'Insubria)
On the Maximum Principle for higher order operators
We discuss a general principle of perturbing higher order operators with lower order derivatives in order to restore the maximum principle in the framework in which it is well known to fail. This is somehow delicate and the main ingredient is a new Harnack-type inequality. We first prove De Giorgi type level estimates for functions in W1,t , with t>2
. This augmented integrability enables us to establish a new Harnack type inequality for functions which do not necessarily belong to De Giorgi's classes as obtained by Di Benedetto-Trudinger for functions in W1,2
. As a consequence, we prove the validity of the strong maximum principle for uniformly elliptic operators of any even order, in fairly general domains and in any dimension, provided either lower order derivatives or inertial effects are taken into account.
12.10.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building, IST - ONLINE)
Marco Fraccaroli (Basque Center for Applied Mathematics)
The time-frequency analysis of the uniform bounds for Hilbert forms
Forms associated with the superposition of bilinear Hilbert transforms appear in many contexts in analysis. For example, developing calculus for pseudo differential operators and studying Cauchy intergrals on Lipschitz curves.
In view of these applications, the question of uniform bounds for such bilinear Hilbert transforms arose. We will explore this problem with a special focus on the multidimensional case. In particular, we will describe the main tool in the time-frequency analysis of such operators, the phase plane projection. This projection concerns the appropriate simultaneous localization of both a function and its Fourier transform to specific regions of the time-frequency support.
This talk is based on joint work with Olli Saari, Christoph Thiele, and Gennady Uraltsev.
26.07.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Dario Mazzoleni (Università di Pavia)
Regularity of the minima of integral shape functionals: the non-degenerate case
We deal with a regularity theorem for the free boundary of solutions to shape optimization problems with integral functionals, for which the energy of a domain $\Omega$ is obtained as the integral of a cost function $j(u,x)$ depending on the solution $u$ of a certain PDE problem on $\Omega$. The main feature of these functionals is that (in general) the minimality of a domain $\Omega$ cannot be translated into a variational problem for a single (real or vector valued) state function, which is the starting point in all the previous regularity results for optimal shapes.
In this paper we focus on the case of affine cost functions $j(u,x)=-g(x)u+Q(x)$, where $u$ is the solution of the PDE $-\Delta u=f$ with Dirichlet boundary conditions. We obtain the Lipschitz continuity and the non-degeneracy of the optimal $u$ from the inwards/outwards optimality of $\Omega$ and then we use the stability of $\Omega$ with respect to variations with smooth vector fields in order to study the blow-up limits of the state function $u$.
By performing a triple consecutive blow-up, we prove the existence of blow-up sequences converging to homogeneous stable solution of the one-phase Bernoulli problem and according to the blow-up limits, we decompose $\partial\Omega$ into a singular and a regular part. In order to estimate the Hausdorff dimension of the singular set of $\partial\Omega$ we give a new formulation of the notion of stability for the one-phase problem, which is preserved under blow-up limits and allows to develop a dimension reduction principle. Finally, by combining a higher order Boundary Harnack principle and a viscosity approach, we prove $C^\infty$ regularity of the regular part of the free boundary (if the data are smooth).
13.07.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building - ONLINE)
Yehyun Kwon (Changwon National University)
Unique continuation for the Schrödinger operators
In this talk, we obtain a complete characterization of the Carleman inequality with the weights $e^{v\cdot x}$ for the polyharmonic operators and deduce unique contin- uation properties for higher order Schr ̈odinger operators with critical potentials. Closely tied toolkits from harmonic analysis are the Stein–Tomas restriction es- timate and the endpoint estimates for the Bochner–Riesz operators of negative indices. This is based on joint work with Eunhee Jeong and Sanghyuk Lee.
04.07.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building)
Alberto Saldaña (Universidad Nacional Autónoma de México)
From competing species to nodal solutions of the Yamabe problem
What is the relationship between wolves fighting and the Poincaré conjecture? These two concepts seem to have nothing in common, but in this talk we will establish a close link by relating three mathematical objects: the Yamabe equation, competitive systems (that model the interaction between two species), and optimal partitions of the sphere. This is joint work with Mónica Clapp and Andrzej Szulkin.
07.06.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building)
Anthony Gauvan (Institut Mathématiques d’Orsay)
Zygmund's problem and axis parallel rectangles
We will discuss a conjecture of Zygmund concerning maximal operators defined on a family of axis parallel rectangles in the Euclidean space. If the historical version of the problem has been disproved by Soria, we will see that the idea behind Zygmund's conjecture may still be true.
In particular, a certain reformulation of the problem has been solved in the Euclidean plane by Stokolos but it remains open in higher dimensions. In the past fews years, different authors (among which D'Aniello, Hagelstei, Oniani, Moonens, Rey, Stokolos etc.) have established sharp weak type estimates in specific settings and their work lend weight to a certain reformulation of Zygmund's conjecture.
We will discuss this problem and in particular, I would like to focus on a specific family of rectangles that exhibits a product structure.
25.05.2023 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building)
João Pedro Ramos (ETH Zürich)
Stability for the sphere packing problem
The celebrated sphere packing problem, a cornerstone of metric geometry, asks what is the densest configuration of points in the Euclidean space such that all points lie at distance at least 2 from each other. A solution to this problem was only known in dimensions $n=1,2,3$, until in 2016 Maryna Viazovska was responsible for a breakthrough result which solved the problem for the additional dimensions $8$ and $24$. She proved, moreover, that the only optimal lattices are the $E_8$ and $\Lambda_{24}$ lattices, in dimensions $n=8$ and $24$, respectively. One may then ask whether any lattice close to attaining optimality in the sphere packing problem is suitably close to those structures, and whether anything can be said for more general (non-lattice) packings.
The purpose of this talk is then to discuss the sphere packing problem, to understand the recent breakthrough by Viazvoska, and to answer the questions in the previous paragraph in the affirmative. We will prove, moreover, a sharp stability result for lattice packings in dimensions $8$ and $24$, and, time-permitting, we shall discuss possible improvements of our results and open questions. This is based on joint work with K. Böröczky and D. Radchenko.
16.03.2023 | 13:30 — 14:30 Europe/Lisbon (IST_room P3.10, Mathematics Building)
Cristian González-Riquelme (CAMGSD, Instituto Superior Técnico)
Continuity and regularity improvement for maximal operators at the derivative level
Maximal operators are a central object in harmonic analysis, The oscillatory behavior of such objects has been an object of study for many authors over the last decades. However, even in the one dimensional case, there are still interesting questions that remain open. In this talk, we will discuss recent developments and open questions about this topic, particularly about the boundedness and continuity for such operators at the derivative level and its relation with some results regarding the regularity improving properties of these operators.
09.03.2023 | 13:30 — 14:30 Europe/Lisbon (IST_room P3.10, Mathematics Building)
Alberto Debernardi Pinos (CIDMA - Universidade de Aveiro)
Gabor orthonormal bases, tiling and periodicity
Given a Gabor orthonormal basis of $L^2(\mathbb{R})$\[\mathcal{G}(g,T,S):=\big\{ g(x-t) e^{2\pi is x}: g\in L^2(\mathbb{R}), \,t\in T,\, s\in S\big\},\]we study periodicity properties of the translation and modulation sets $T$ and $S$. In particular, we show that if the window function $g$ is compactly supported, then $T$ and $S$ must be periodic sets, i.e., of the form\[T = a\mathbb{Z}+ \{t_1,\ldots,t_n\}, \qquad S = b\mathbb{Z} + \{s_1,\ldots,s_m\}.\]To achieve this, we first obtain a result of independent interest: if the system $\mathcal{G}(g,T,S)$ is an orthonormal basis of $L^2(\mathbb{R})$, then both $|g|^2$ and $|\widehat{g}|^2$ tile $\mathbb{R}$ by translations (when translated along the sets $T$ and $S$, respectively), and moreover,\[\sum_{t\in T} |g(x-t)|^2=D(T), \qquad \sum_{s\in S} |\widehat{g}(x-s)|^2=D(S), \qquad \text{a.e. }x\in \mathbb{R},\]where $D(\Lambda)$ denotes the uniform density of a set $\Lambda\subset \mathbb{R}$.
Partial results towards the Liu-Wang conjecture are also obtained.
This talked in based on a joint work with Nir Lev (Bar-Ilan University, Israel)
02.02.2023 | 13:30 — 14:30 Europe/Lisbon (room 6.2.33, Faculty of Sciences, ULisboa)
Edgard Pimentel (Universidade de Coimbra)
A glimpse on the regularity theory for fully nonlinear equations
We discuss the very basics of fully nonlinear elliptic equations, in close connection with regularity results. The latter include the celebrated Krylov-Safonov and Evans-Krylov theorems and the fundamental developments in Caffarelli’s theory. We also put forward more recent advances, such as the smoothness of flat solutions and the partial regularity result. In face of this panorama, we present some of our recent contributions to the theory. It covers fractional regularity estimates, the use of the Harnack approach and the connection with free boundary problems. We conclude with a discussion of a few open questions and their main challenges.
19.01.2023 | 13:30 — 14:30 Europe/Lisbon (room 6.2.33, Faculty of Sciences, ULisboa)
Pasquale Candito (Universidade de Reggio Calabria)
Existence results for nonlinear differential problems
The main aim of the talk is to discuss some existence results for nonlinear differential problems. First, a survey on existence results for boundary value problems (Dirichlet, Neumann, Periodic) obtained by using a coincidence point theorem for sequentially continuous mapping, is given. Next, a recent result, established combining a priori bounds, difference, truncations techniques and variational methods, for a two-point boundary value problem, is presented.
12.01.2023 | 13:30 — 14:30 Europe/Lisbon ONLINE
Jiawei Li (University of Edinburgh)
On distributions of random vorticity and velocity fields in turbulent flows
In this talk, we are interested in the vorticity and velocity random fields of turbulent flows. I will introduce the evolution equations for probability density functions of these random fields under some conditions on the flow. I will also talk about several methods we used to solve these PDF PDEs numerically. Based on joint works with Zhongmin Qian and Mingrui Zhou.
17.11.2022 | 13:30 — 14:30 Europe/Lisbon (room P3.10, Mathematics Building)
Giuseppe Negro (CAMGSD, Instituto Superior Técnico)
Explicit solutions to the cubic wave equation
We construct a two-parameter family of solutions to the focusing cubic wave equation in $\mathbb{R}^{1+3}$. Depending on the values of the parameters, these solutions either scatter to linear ones, blow-up in finite time, or exhibit a new type of unstable behaviour that acts as a threshold between the other two. We further prove that the blow-up behaviour is stable and we characterize the threshold behaviour precisely, both pointwise and in Sobolev sense.
Joint work with Thomas Duyckaerts (Sorbonne Paris Nord).
13.09.2022 | 14:00 — 15:00 Europe/Lisbon — Room P3.10, Mathematics Building, Instituto Superior Técnico
Jonathan Bennett (University of Birmingham)
The nonlinear Brascamp-Lieb inequality and applications
The Brascamp-Lieb inequality is a broad generalisation of many well-known multilinear inequalities in analysis, including the multilinear Hölder, Loomis-Whitney and sharp Young convolution inequalities. There is by now a rich theory surrounding this classical inequality, along with applications in convex geometry, harmonic analysis, partial differential equations, number theory and beyond. In this talk we present a certain nonlinear variant of the Brascamp-Lieb inequality, placing particular emphasis on some of its applications. Most of this is joint work with Stefan Buschenhenke, Neal Bez, Michael Cowling and Taryn Flock.
21.07.2022 | 14:00 — 15:00 Europe/Lisbon (room 6.2.33, FCUL)
Jörg Wolf (Department of Mathematics, Chung-Ang University, Seoul)
Existence of weak solutions the equations of a non-Newtonian fluid with non standard growth
We consider the equations of a non-Newtonian incompressible fluid in a general time space cylinder $Q_{T}= \Omega \times (0,T) \subset \mathbb{R}^{n} \times \mathbb{R}, n \geq 2$. We assume that the rheology of the fluid is changing with respect to time and space and satisfies for each $(x,t) \in Q_{T}$ the associated power law $ |D|^{p(x,t) } D $. Under the assumption that $ \frac{2n}{n+2} < p_{0} \le p(x,t) \leq p_{1} < +\infty$ and the set of discontinuity of $p$ is closed and of measure zero we show the existence of a weak solution to the corresponding equations of PDEs for any given initial velocity in $L^{2}_{\sigma } (\Omega) $. Joint work with Prof. H-O. Bae (Ajou University, Suwon).
17.06.2022 | 11:00 — 12:00 Europe/Lisbon (room 6.2.33, FCUL)
Sergei Kuksin (Univ. Paris VII)
Kolmogorov theory of turbulence and a rigorous theory of one-dimensional turbulence
My talk is a review of the results on turbulence in the 1d Burgers equation, presented in my book One-dimensional turbulence and stochastic Burgers equation (2021), written jointly with A. Boritchev. Namely, I will talk about the viscous Burgers equation on a circle, perturbed by a random force which is smooth in $x$ and white in time $t$, and explain that Sobolev norms of its solutions admit upper and lower estimates, which are asymptotically sharp as the viscosity goes to zero. This assertion allows to derive for solutions of the equation results, which are rigorous analogies of the main predictions of the Kolmogorov theory of turbulence. Namely, of the Kolmogorov 2/3-law for increments of the turbulent velocity-fields and of the Kolmogorov-Obukhov 5/3-law for the energy spectrum of turbulence (I will explain these laws). The results were non-rigorously obtained by physicists in 1990s (and earlier by J. Burgers in 1948, even more heuristically).
15.06.2022 | 14:00 — 15:00 Europe/Lisbon (room P3.10, Mathematics Building, IST)
João Pedro Ramos (ETH Zurich)
Time-frequency localisation operators, their eigenvalues and relationship to elliptic PDE
In the classical realm of time-frequency analysis, a classical object of interest is the short-time Fourier transform of a function. This object is a modified Fourier transform of a signal $f(x),$ modified by a certain 'window function', in order to make joint time-frequency analysis of functions more feasible.
Since the pioneering work of Daubechies, time-frequency localisation operators have been of extreme importance in that analysis. These are defined through $V^* 1_{\Omega} V f = P_{\Omega} f,$ where $V$ denotes the short-time Fourier transform with some fixed window. These operators seek to measure how much a function concentrates in the time-frequency plane, and thus the study of their eigenvalues and eigenfunctions is intimately connected to the previous questions.
In this talk, we will explore the case of a Gaussian window function $\varphi(x) = e^{-\pi x^2}$, and the operators thus obtained. We will discuss some classical and recent results on domains of maximal time-frequency concentration, their eigenvalues, and inverse problems associated with such properties. During this investigation, we shall see that many of these problems possess some rather unexpected connections with overdetermined elliptic boundary value problems and free boundary problems in general. This is based on recent joint work with Paolo Tilli.
08.06.2022 | 14:00 — 15:00 Europe/Lisbon (room P3.10, Mathematics Building, IST)
Luccas Campos (Universidade Federal de Minas Gerais)
The role of the inhomogeneity on non-radial scattering for nonlinear Schrödinger equations
The concentration-compactness-rigidity method, pioneered by Kenig and Merle, has become standard in the study of global well-posedness and scattering in the context of dispersive and wave equations. Albeit powerful, it requires building some heavy machinery in order to obtain the desired space-time bounds. In this talk, we present a simpler method, based on Tao's scattering criterion and on Dodson-Murphy's Virial/Morawetz inequalities, first proved for the 3d cubic nonlinear Schrödinger (NLS) equation.
Tao's criterion is, in some sense, universal, and it is expected to work in similar ways for dispersive problems. On the other hand, the Virial/Morawetz inequalities need to be established individually for each equation, as they rely on monotonicity formulae. To treat the non-radial case, the original Dodson-Murphy's approach for the NLS equation relies heavily on the conservation of momentum and on the Galilean invariance. This poses an obstacle for equations which do not present these features. We show how to circumvent this problem in the case of the inhomogeneous NLS, by exploiting the decay of the nonlinearity, making it possible to drop the radiality assumption. The approach is versatile, as it can be shown to work in the energy-subcritical setting for different spatially decaying nonlinearities, as well as for higher-order equations.
01.06.2022 | 14:00 — 15:00 Europe/Lisbon
Rainer Mandel (Karlsruher Institut für Technologie)
New Gagliardo-Nirenberg inequalities and applications to biharmonic NLS
We present new existence results for nontrivial solutions of some biharmonic Nonlinear Schrödinger equation in $\mathbb{R}^N$ that are based on a constrained minimization approach. Here the main difficulty comes from the fact that spherical rearrangements need not decrease the energy so that more sophisticated arguments are needed to overcome the lack of compactness. A new and intrisically motivated tool is given by a new class of Gagliardo-Nirenberg inequalities where, essentially, the Laplacian in the classical Gagliardo-Nirenberg inequality is replaced by the Helmholtz operator. Having explained the relevance of such inequalities for our analysis, we comment on their proofs and related questions from Harmonic Analysis. Finally, we shall mention a symmetry-breaking phenomenon related to our results that was recently observed by Lenzmann and Weth. Accordingly, the talk covers topics from the Calculus of Variations as well as Harmonic Analysis or, more specifically, Fourier Restr iction Theory.
25.05.2022 | 14:00 — 15:00 Europe/Lisbon (room C6.2.33, FCUL)
Alberto Saldaña (Universidad Nacional Autónoma de México)
An introduction to the logarithmic Laplacian: what it is and what are some of its applications.
Fractional derivatives are commonly used to model a variety of phenomena, but… what does it mean to have a logarithmic derivative? And what would it be used for?
In this talk we focus on the logarithmic Laplacian, a pseudodifferential operator that appears as a first order expansion of the fractional Laplacian of order 2s as s goes to zero. This operator can also be represented as an integrodifferential operator with a zero order kernel.
We will discuss how this operator can be used to study the behavior of linear and nonlinear fractional problems in the small order limit. This analysis will also reveal a deep and interesting mathematical structure behind the set of solutions of Dirichlet logarithmic problems.
18.05.2022 | 14:00 — 15:00 Europe/Lisbon (Room P3.10, Mathematics Building (IST))
Joris Roos (University of Edinburgh)
Spherical maximal functions and fractal dimensions of dilation sets
In this talk we will consider spherical maximal operators in Euclidean space with a supremum taken over a given dilation set. It turns out that the sharp $L^p$ improving properties of such operators are closely related to fractal dimensions of the dilation set such as the Minkowski and Assouad dimensions.
At the center of the talk will be a simple characterization of the closed convex sets which can occur as closure of the sharp $L^p$ improving region of such a maximal operator.
This is joint work with Andreas Seeger. Time permitting, we will also discuss some ongoing work and further directions.
11.05.2022 | 14:00 — 15:00 Europe/Lisbon Room P3.10, Mathematics Building (Instituto Superior Técnico)
Angela Pistoia (Università di Roma "La Sapienza")
Segregated solutions for some nonlinear Schrödinger systems
I will focus on the existence and multiplicity of positive non-radial solutions for some systems of Schrödinger equations in a weak fully attractive or repulsive regime in presence or in absence of an external radial trapping. The results have been obtained in collaboration with Haixia Chen (Central China Normal University, Wuhan), Maria Medina (Universidad Autonoma de Madrid ) and Giusi Vaira (Università di Bari).
04.05.2022 | 14:00 — 15:00 Europe/Lisbon Room P3.10, Mathematics Building (IST)
Delia Schiera (CAMGSD, Instituto Superior Técnico)
Positive solutions to a nonlinear Choquard equation with symmetry
I will present some existence results for a class of Choquard equations in which the potential has a positive limit at infinity and satisfies suitable decay assumptions. Also, it is taken invariant under the action of a closed subgroup of linear isometries of $\mathbb{R}^N$. As a consequence, the positive solution found is invariant under the same action. We investigate superlinear, linear and sublinear nonlinearities, and we take into account an arbitrary number of bumps.
Joint work together with Liliane Maia and Benedetta Pellacci.
20.04.2022 | 14:00 — 15:00 Europe/Lisbon
Jean Van Schaftingen (Université Catholique de Louvain)
Ginzburg-Landau functionals on planar domains for a general compact vacuum manifold
Ginzburg-Landau type functionals provide a relaxation scheme to construct harmonic maps in the presence of topological obstructions. They arise in superconductivity models, in liquid crystal models (Landau-de Gennes functional) and in the generation of cross-fields in meshing. For a general compact manifold target space we describe the asymptotic number, type and location of singularities that arise in minimizers. We cover in particular the case where the fundamental group of the vacuum manifold in nonabelian and hence the singularities cannot be characterized univocally as elements of the fundamental group. We obtain similar results for \(p\)-harmonic maps with \(p\) going to \(2\).
The results unify the existing theory and cover new situations and problems.
This is a joint work with Antonin Monteil (Paris-Est Créteil, France), Rémy Rodiac (Paris-€“Saclay, France) and Benoit Van Vaerenbergh (UCLouvain).
07.04.2022 | 14:00 — 15:00 Europe/Lisbon
Diego Moreira (Universidade Federal do Ceará)
Semiconvex Supersolutions to Fully Nonlinear PDEs
In this talk, we discuss some results in collaboration with Alessio Figalli (ETH Zurich) and Ederson Braga (UFC) about the optimal regularity for semi-convex supersolutions to fully nonlinear PDEs. This extends the Caffarelli Kohn-Nirenberg-Spruck apriori estimates in the 80s. It has also some implications for improving regularity for Sobolev embeddings in the presence of some (general) convexity properties. If time permits, some ideas of the proof and open problems will be discussed.
To be held online at: https://videoconf-colibri.zoom.us/j/86873402503
Online access password: lisbonwade
06.04.2022 | 14:00 — 15:00 Europe/Lisbon — room 6.2.33, Faculty of Sciences of the Universidade de Lisboa
Wladimir Neves (Universidade Federal do Rio de Janeiro)
Solvability of the Fractional Hyperbolic Keller-Segel System
We study a new nonlocal approach to the mathematical modelling of the Chemotaxis problem, which describes the random motion of a certain population due a substance concentration. Considering the initial- boundary value problem for the fractional hyperbolic Keller-Segel model, we prove the solvability of the problem. The solvability result relies mostly on fractional calculus and kinetic formulation of scalar conservation laws.
To be held online at: https://videoconf-colibri.zoom.us/j/6751812409
No password needed for this session.
31.03.2022 | 16:30 — 17:30 Europe/Lisbon — room 6.2.33, Faculty of Sciences of the Universidade de Lisboa
Jaqueline Mesquita (Universidade de Brasília)
Linearized instability for neutral FDEs with state-dependent delays
In this talk, we present a linearized instability principle for neutral FDEs with state-dependent delays. This is a joint work with Bernhard Lani-Wayda from Justus-Liebig Universität, Giessen.
18.11.2021 | 14:00 — 15:00 Europe/Lisbon
Giovanni Bellettini, ICTP and Università di Siena
On a conjecture of De Giorgi on the first variation of the Modica-Mortola functional
We shall discuss some (not so recent) results on a 1991 conjecture of De Giorgi concerning the Gamma limit of the square norm of the first variation of the Modica-Mortola functionals, and its relation with the Willmore functional.
04.11.2021 | 14:00 — 15:00 Europe/Lisbon
Kelei Wang, Wuhan University
Regularity of transition layers in Allen-Cahn equation
In this talk I will survey the regularity theory for transition layers in singularly perturbed Allen-Cahn equation, from zeroth order regularity to second order one. Some applications of this regularity theory will also be discussed, including De Giorgi conjecture, classification of finite Morse index solutions and construction of minimal hypersurfaces by Allen-Cahn approximation.
21.10.2021 | 14:00 — 15:00 Europe/Lisbon
Simone Dovetta, Università degli Studi di Roma "La Sapienza"
Action versus energy ground states in nonlinear Schrödinger equations
The talk investigates the relations between normalized critical points of the nonlinear Schrödinger energy functional and critical points of the corresponding action functional on the associated Nehari manifold.
First, we show that the ground state levels are strongly related by the following duality result: the (negative) energy ground state level is the Legendre—Fenchel transform of the action ground state level. Furthermore, whenever an energy ground state exists at a certain frequency, then all action ground states with that frequency have the same mass and are energy ground states too. We see that the converse is in general false and that the action ground state level may fail to be convex. Next we analyze the differentiability of the ground state action level and we provide an explicit expression involving the mass of action ground states. Finally we show that similar results hold also for local minimizers, and we exhibit examples of domains where our results apply.
The matter of the talk refers to joint works with Enrico Serra and Paolo Tilli.
14.10.2021 | 16:00 — 17:00 Europe/Lisbon
Juraj Földes, University of Virginia
Stochastic approach to boundary regularity of hypoelliptic PDEs
We will discuss the almost sure behavior of solutions of stochastic differential equations(SDEs) as time goes to zero. Our main general result establishes a functional law of the iterated logarithm (LIL) that applies in the setting of SDEs with degenerate noise satisfying the weak Hormander condition. We will introduce large deviations to provide some details of proofs. Furthermore, we apply the stochastic results to the problem of identifying regular points for hypoelliptic diffusions and obtain criteria for well posedness of degenerate equations.
This is a joint work with David Herzog and Marco Carfagnini.
27.05.2021 | 14:00 — 15:00 Europe/Lisbon
Julio D. Rossi (Universidad de Buenos Aires)
Non Linear Mean Value Properties for Monge-Ampère Equations
In recent years there has been an increasing interest in whether a mean value property, known to characterize harmonic functions, can be extended in some weak form to solutions of nonlinear equations. This question has been partially motivated by the surprising connection between Random Tug-of-War games and the normalized $p-$Laplacian discovered some years ago, where a nonlinear asymptotic mean value property for solutions of a PDE is related to a dynamic programming principle for an appropriate game.
Our goal in this talk is to show that an asymptotic nonlinear mean value formula holds for the classical Monge-Ampère equation.
Joint work with P. Blanc (Jyvaskyla), F. Charro (Detroit), and J.J. Manfredi (Pittsburgh).
20.05.2021 | 15:00 — 16:00 Europe/Lisbon
Mariana Smit Vega Garcia (Western Washington University)
Regularity of almost minimizers with free boundary
We study almost minimizer for functionals that yield a free boundary, as in the work of Alt-Caffarelli and Alt-Caffarelli-Friedman. The almost minimizing property can be understood as the defining characteristic of a minimizer in a problem that explicitly takes noise into account. In this talk, we discuss the regularity of almost minimizers to energy functionals with variable coefficients. This is joint work with Guy David, Max Engelstein & Tatiana Toro.
** Please note that the seminar is one hour later than the usual time **
13.05.2021 | 14:00 — 15:00 Europe/Lisbon
Simone Di Marino (Università di Genova)
An entropy minimization approach to second-order variational mean field games
The use of the entropic regularization and the relative Sinkhorn algorithm in order to calculate optimal transport problems is nowadays widely used.
We propose a new viewpoint on application of this regularization technique to variational mean-field games with quadratic Hamiltonian. In particular we focus on the fact that this regularization amounts to solving second order variational mean-field games, dropping in particular the requirement for the regularization parameter to be small, whenever we are interested in the case with diffusion.
Based on a joint work with J.-D. Benamou, G. Carlier and L. Nenna.
06.05.2021 | 14:00 — 15:00 Europe/Lisbon
Didier Pilod (University of Bergen)
Global well-posedness and scattering for the Dysthe equation in ![]()
The Dysthe equation is a higher order approximation of the water waves system in the modulation (Schrödinger) regime and in the infinite depth case. After reviewing the derivation of the Dysthe and related equations, we will focus on the initial-value problem. We prove a small data global well-posedness and scattering result in the critical space ![]() . This result is sharp in view of the fact that the flow map cannot be
. This result is sharp in view of the fact that the flow map cannot be ![]() continuous below
continuous below ![]() .
.
Our analysis relies on linear and bilinear Strichartz estimates in the context of the Fourier restriction norm method. Moreover, since we are at a critical level, we need to work in the framework of the atomic space ![]() and its dual
and its dual ![]() of square bounded variation functions.
of square bounded variation functions.
We also prove that the initial-value problem is locally well-posed in ![]() ,
, ![]()
Our results extend to the finite depth version of the Dysthe equation.
This talk is based on a joint work with Razvan Mosincat (University of Bergen) and Jean-Claude Saut (Université Paris-Saclay).
29.04.2021
14:00 — 15:00 Europe/Lisbon
Jan Mass (IST Austria)
Homogenisation of discrete dynamical optimal transport
Many stochastic systems can be viewed as gradient flow ('steepest descent') in the space of probability measures, where the driving functional is a relative entropy and the relevant geometry is described by a dynamical optimal transport problem. In this talk we focus on these optimal transport problems and describe recent work on the limit passage from discrete to continuous.
Surprisingly, it turns out that discrete transport metrics may fail to converge to the expected limit, even when the associated gradient flows converge. We will illustrate this phenomenon in examples and present a recent homogenisation result.
This talk is based on joint work with Peter Gladbach, Eva Kopfer, and Lorenzo Portinale.
******
15:00 — 16:00 Europe/Lisbon
Max Fathi (Université de Paris)
A proof of the Caffarelli contraction theorem via entropic interpolation
The Caffarelli contraction theorem states that optimal transport maps (for the quadratic cost) from a Gaussian measure onto measures that satisfy certain convexity properties are globally Lipschitz, with a dimension-free estimate. It has found many applications in probability, such as concentration and functional inequalities. In this talk, I will present an alternative proof, using entropic interpolation and variational arguments. Joint work with Nathael Gozlan and Maxime Prod'homme.
22.04.2021 | 14:00 — 15:00 Europe/Lisbon
Juan Luis Vázquez (Universidad Autónoma de Madrid)
The theory of fractional p-Laplacian equations
We consider the time-dependent fractional $p$-Laplacian equation with parameter $p>1$ and fractional exponent $0<$ $s<1$. It is the gradient flow corresponding to the Gagliardo fractional energy. Our main result is the asymptotic behavior of solutions posed in the whole Euclidean space, which is given by a kind of Barenblatt solution whose existence relies on delicate analysis. We will concentrate on the sublinear or “fast” regime, $1<$ $p<2$, since it offers a richer theory. Fine bounds in the form of global Harnack inequalities are obtained as well as solutions having strong point singularities (Very Singular Solutions) that exist for a very special parameter interval. They are related to fractional elliptic problems of nonlinear eigenvalue form. Extinction phenomena are discussed.
15.04.2021
14:00 — 15:00 Europe/Lisbon
Nicola Fusco (Università di Napoli "Federico II")
Asymptotic stability for the gradient flow of some nonlocal energies
I will start by discussing some recent results on the asymptotic stability of the $H^{-1}$-gradient flow of the perimeter, the so called surface diffusion. Then I will consider the $H^{-1}$-gradient flow of some energy functionals given by the area of an interface plus a non local volume term.
This is a joint work with E. Acerbi, V. Julin and M. Morini
******
15:00 — 16:00 Europe/Lisbon
Riccardo Scala (Università degli Studi di Siena)
Nonlocality features of the area functional and the Plateau problem
We briefly discuss the definition of relaxation of the area functional. The relaxed area functional, denoted by $A$, extends the classical area functional, which, for any "regular" map $v:U\subset \mathbb{R}^n\rightarrow \mathbb{R}^N$ evaluates the $n$-dimensional area of its graph over $U$. The problem of determining the domain and the expression of $A$ is open in codimension greater than 1. Specifically, this relaxed functional turns out to be nonlocal and cannot be expressed by an integral formula. We discuss how it is related to classical and nonclassical versions of the Plateau problem. As a main example, we try to understand what is the relaxed graph of the function $x/|x|$, a question that surprisingly remained open for decades.
Next webinar | https://wade.ulisboa.pt/
To be held online at: https://videoconf-colibri.zoom.us/j/85036762376
Online access password: lisbonwade
08.04.2021 | 14:00 — 15:00 Europe/Lisbon
Valeria Chiadò Piat (Politecnico di Torino)
An extension theorem from connected sets and homogenization of non-local functionals
Extensions operators are a classical tool to provide uniform estimates and gain compactness in the homogenization of integral functionals over perforated domains. In this talk we discuss the case of non-local functionals. The results are obtained in collaboration with Andrea Braides and Lorenza D'Elia.
01.04.2021 | 14:00 — 15:00 Europe/Lisbon
Grégoire Allaire (CMAP, École Polytechnique)
Some problems and some solutions in shape and topology optimization of structures built by additive manufacturing
Additive manufacturing (or 3-d printing) is a new exciting way of building structures without any restriction on their topologies. However, it comes with its own difficulties or new issues. Therefore, it is a source of many interesting new problems for optimization. I shall discuss two of them and propose solutions to these problems, but there is still a lot of room for improvement!
First, additive manufacturing technologies are able to build finely graded microstructures (called lattice materials). Their optimization is therefore an important issue but also an opportunity for the resurrection of the homogenization method ! Indeed, homogenization is the right technique to deal with microstructured materials where anisotropy plays a key role, a feature which is absent from more popular methods, like SIMP. I will describe recent work on the topology optimization of these lattice materials, based on a combination of homogenization theory and geometrical methods for the overall deformation of the lattice grid.
Second, additive manufacturing, especially in its powder bed fusion technique, is a very slow process because a laser beam must travel along a trajectory, which covers the entire structure, to melt the powder. Therefore, the optimization of the laser path is an important issue. Not only do we propose an optimization strategy for the laser path, but we couple it with the usual shape and topology optimization of the structure. Numerical results show that these two optimizations are tightly coupled.
This is a joint work with many colleagues, including two former PhD students, P. Geoffroy-Donders and M. Boissier.
25.03.2021 | 11:00 — 12:00 Europe/Lisbon
Yukihiko Nakata (Aoyama Gakuin University, Tokyo)
Period two solution for a class of distributed delay differential equations
We consider a periodic solution for a class of distributed delay differential equations.
A period two solution for distributed delay differential equations, where the period is twice the maximum delay, is shown to satisfy a Hamiltonian system of ordinary differential equations, from which we can construct the period two solution for the distributed delay differential equation.
The idea is based on Kaplan & Yorke (1974, JMAA) for a discrete delay differential equation. We present distributed delay differential equations that have periodic solutions expressed in terms of the Jacobi elliptic functions.
18.03.2021 | 14:00 — 15:00 Europe/Lisbon
Gabriele Benomio (Princeton University)
A new instability for higher dimensional black holes
The dynamics of solutions to the Einstein equations is richer in dimensions higher than . In contrast with the classical stability of stationary, asymptotically flat black hole solutions in dimensions, some families of higher dimensional black holes suffer from dynamical instabilities. I will discuss a subtle instability affecting a wide class of higher dimensional black holes which has not been previously observed in the literature. This new instability is, in a sense, more fundamental than the other known instability phenomena in higher dimensions and can be related to a precise geometric property of the class of spacetimes considered.
******
18.03.2021 | 15:00 — 16:00 Europe/Lisbon
Shrish Parmeshwar (University of Bath)
Global-in-Time Solutions to the N-Body Euler-Poisson System
We investigate the -Body compressible Euler-Poisson system, modelling multiple stars interacting with each other via Newtonian gravity. If we prescribe initial data so that each star expands indefinitely, one might expect that two of them will collide in finite time due to their expansion, and the influence of gravity. In this talk we show that there exists a large family of initial positions and velocities for the system such that each star can expand for all time, but no two will touch in finite time. To do this we use scaling mechanisms present in the compressible Euler system, and a careful analysis of how the gravitational interaction between stars affects their dynamics.
11.03.2021 | 14:00 — 15:00 Europe/Lisbon
Bruno Premoselli (Université Libre de Bruxelles)
Towers of bubbles for Yamabe-type equations in dimensions larger than 7
In this talk we consider perturbations of Yamabe-type equations on closed Riemannian manifolds. In dimensions larger than 7 and on locally conformally flat manifolds we construct blowing-up solutions that behave like towers of bubbles concentrating at a critical point of the mass function. Our result does not assume any symmetry on the underlying manifold.
We perform our construction by combining finite-dimensional reduction methods with a linear blow-up analysis in order to sharply control the remainder of the construction in strong spaces. Our approach works both in the positive and sign-changing case. As an application we prove the existence, on a generic bounded open set of $\mathbb{R}^n$, of blowing-up solutions of the Brézis-Nirenberg equation that behave like towers of bubbles of alternating signs.
04.03.2021 | 14:00 — 15:00 Europe/Lisbon
Bozhidar Velichkov (Università di Pisa)
Vectorial free boundary problems
The vectorial Bernoulli problem is a variational free boundary problem involving the Dirichlet energy of a vector-valued function and the measure of its support. It is the vectorial counterpart of the classical one-phase Bernoulli problem, which was first studied by Alt and Caffarelli in 1981.
In this talk, we will discuss some results on the regularity of the vectorial free boundaries obtained in the last years by Caffarelli-Shahgholian-Yeressian, Kriventsov-Lin, Mazzoleni-Terracini-V., and Spolaor-V.. Finally, we will present some new results on the rectifiability of the singular set obtained in collaboration with Guido De Philippis, Max Engelstein and Luca Spolaor.
***
04.03.2021 | 15:00 — 16:00 Europe/Lisbon
Dario Mazzoleni (Università di Pavia)
Regularity of the optimal sets for the second Dirichlet eigenvalue
First of all, we recall the basic notions and results concerning shape optimization problems for the eigenvalues of the Dirichlet Laplacian.
Then we focus on the study of the regularity of the optimal shapes and on the link with the regularity of related free boundary problems.
The main topic of the talk is the regularity of the optimal sets for a "degenerate'" functional, namely the second Dirichlet eigenvalue in a box. Given an open and bounded set of class we consider the following shape optimization problem, for ,
where denotes the second eigenvalue of the Dirichlet Laplacian on .
In this talk we show that any optimal set for (1) is equivalent to the union of two disjoint open sets, , which are regular up to a (possibly empty) closed singular set of Hausdorff dimension at most , which is contained in the one-phase free boundaries.
In particular, we are able to prove that the set of two-phase points, that is, , is contained in the regular set.
This is a joint work with Baptiste Trey and Bozhidar Velichkov.
To be held online at: https://videoconf-colibri.zoom.us/j/81421184299
Online access password: lisbonwade
25.02.2021 | 14:00 — 15:00 Europe/Lisbon
Boyan Sirakov (PUC - Rio)
The Vázquez maximum principle and the Landis conjecture for elliptic PDE with unbounded coefficients
In this joint work with P. Souplet we develop a new, unified approach to the following two classical questions on elliptic PDE:
(i) the strong maximum principle for equations with non-Lipschitz nonlinearities; and
(ii) the at most exponential decay of solutions in the whole space or exterior domains.
Our results apply to divergence and nondivergence operators with locally unbounded lower-order coefficients, in a number of situations where all previous results required bounded ingredients. Our approach, which allows for relatively simple and short proofs, is based on a (weak) Harnack inequality with optimal dependence of the constants in the lower-order terms of the equation and the size of the domain, which we establish.
18.02.2021 | Thursday | 14:00 — 15:00 Europe/Lisbon
Paolo Gidoni (Czech Academy of Sciences)
A vanishing inertia analysis for finite dimensional rate-independent systems and an application to soft crawlers
The quasistatic limit is a convenient approximation in the modelling of several (suitable) mechanical systems, when the evolution occurs at a sufficiently slow time-scale. In this talk we discuss the validity of the quasistatic approximation in finite-dimensional rate-independent systems via a vanishing-inertia asymptotic analysis of dynamic evolutions. More precisely, we show the uniform convergence of dynamic solutions to the quasistatic one, employing the concept of energetic solution. Our work is motivated by the application to a family of models for biological and bio-inspired crawling locomotion. Hence a part of the seminar will focus on modelling: we will discuss how soft crawlers can be effectively described in our theoretical framework and briefly survey the relevance, or lack thereof, of inertia in some locomotion strategies. By a technical point of view, our application requires time-dependence of the dissipation potential and translation invariance of the potential energy.
11.02.2021 | Thursday | 14:00 — 15:00 Europe/Lisbon
Harbir Antil (George Mason University)
Fractional PDEs: Control, Numerics, and Applications
Fractional calculus and its application to anomalous diffusion has recently received a tremendous amount of attention. In complex/heterogeneous material mediums, the long-range correlations or hereditary material properties are presumed to be the cause of such anomalous behavior. Owing to the revival of fractional calculus, these effects are now conveniently modeled by fractional-order differential operators and the governing equations are reformulated accordingly. Similarly, the potential of fractional operators has been harnessed in various scientific domains like geophysical electromagnetics, imaging science, deep learning, etc.
In this talk, fractional operators will be introduced and both linear and nonlinear, fractional-order differential equations will be discussed. New notions of optimal control and optimization under uncertainty will be presented. Several applications from geophysics, imaging science, and deep learning will be presented.
04.02.2021 | Thursday | 14:00 — 15:00 Europe/Lisbon
Yvan Martel (École Polytechnique)
On the asymptotic stability of kinks for (1+1)-scalar field models
The talk concerns stability properties of kinks for (1+1)-dimensional nonlinear scalar field models of the form
![]()
We establish a simple and explicit sufficient condition on the potential for the asymptotic stability of a given moving or standing kink.
We present applications of the criterion to some models of the Physics literature.
Work in collaboration with Michał Kowalczyk, Claudio Muñoz and Hanne Van Den Bosch. See also the related work with Michał Kowalczyk and Claudio Muñoz.
28.01.2021 | Thursday | 14:00 — 15:00 Europe/Lisbon
Enrico Serra (Politecnico di Torino)
NLS ground states on metric trees: existence results and open questions
We consider the minimization of the NLS energy on a metric tree, either rooted or unrooted, subject to a mass constraint. With respect to the same problem on other types of metric graphs, several new features appear, such as the existence of minimizers with positive energy, and the emergence of unexpected threshold phenomena. We also study the problem with a radial symmetry constraint that is in principle different from the free problem due to the failure of the Polya-Szego inequality for radial rearrangements. A key role is played by a new Poincaré inequality with remainder.
21.01.2021 | 14:00 — 15:00 Europe/Lisbon
Charles Collot, (Cergy Paris Université)
On the stability of equilibria for infinitely many particles
We study the evolution of a system of particles. Instead of the usual Hartree equation for density matrices, we consider the following equivalent model, proposed by de Suzzoni, of a Hartree type equation but for a random field:
![]()
Above, ![]() is a time-dependent random field, a pair interaction potential, *the convolution product and
is a time-dependent random field, a pair interaction potential, *the convolution product and ![]() the expectation. This equation admits equilibria which are random Gaussian fields whose laws are invariant by time and space translations. They are hence not localised and represent an infinite number of particles. We give a stability result under certain hypotheses, by showing that small perturbations scatter as
the expectation. This equation admits equilibria which are random Gaussian fields whose laws are invariant by time and space translations. They are hence not localised and represent an infinite number of particles. We give a stability result under certain hypotheses, by showing that small perturbations scatter as ![]() to linear waves. This is joint work with de Suzzoni.
to linear waves. This is joint work with de Suzzoni.
14.01.2021 | 10:00 — 12:00 Europe/Lisbon
Serena Dipierro & Enrico Valdinoci (University of Western Australia)
Nonlocal Minimal Surfaces: interior regularity, boundary behavior and stickiness phenomena
Surfaces which minimize a nonlocal perimeter functional exhibit quite different behaviors than the ones minimizing the classical perimeter. We will investigate some structural properties of nonlocal minimal surfaces both in the interior of a given domain and in the vicinity of its boundary. Among these peculiar features, an interesting property, which is also in contrast with the pattern produced by the solutions of linear equations, is given by the capacity, and the strong tendency, of adhering at the boundary. We will also discuss this phenomenon and present some recent results.
(These are two consecutive talks: Part I is given by Serena Dipierro, Part II by Enrico Valdinoci)
07.01.2021 | 16:30 — 17:30 Europe/Lisbon
Nilima Nigam (Simon Fraser University)
Boundary integral strategies for the Steklov eigenproblem
In Steklov eigenproblems for elliptic operators, the spectral parameter links boundary traces of eigenfunctions to traces of the Neumann data. It is natural, therefore, to reformulate such eigenproblems in terms of boundary integral operators, which allow for nonsmooth boundaries. In this talk we describe such strategies in the context of Steklov problems for the Laplacian as well as the Helmholtz operator, and their use in studying questions arising in spectral geometry.
17.12.2020 | 14:30 — 15:30 Europe/Lisbon
Svetlana Roudenko (Florida International University)
Zakharov-Kuznetsov equation: toward soliton resolution
We consider Zakharov-Kuznetsov (ZK) equation, which is a higher-dimensional version of the Korteweg-de Vries (KdV) equation, and investigate the dynamics of solutions, especially questions about the soliton stability. We first discuss the situation in two dimensions, in particular, the instability of solitons in the 2d cubic (critical) ZK equation, which leads to blow-up. Then we consider the 3d quadratic ZK equation, originally introduced by Zakharov and Kuznetsov in early 1970's, and discuss the asymptotic stability of solitons. We will also show numerical findings on the formation of solitons and radiation in this equation. This talk will be based on joint works with L.G. Farah, J. Holmer, C. Klein, N. Stoilov, K. Yang.
10.12.2020 | 14:30 — 15:30 Europe/Lisbon
Qiao Huang (GFM, Universidade de Lisboa)
Stochastic Cucker-Smale model: collision-avoidance and flocking
In this talk, we consider the Cucker-Smale flocking model involving both singularity and noise. We first show the local strong well-posedness for the system, in which the communication weight is locally Lipschitz beyond the origin. Then, for the special case that the communication weight has a strong singularity at the origin, we establish the global well-posedness by showing the finite time collision-avoidance. Finally, we study the large time behavior of the system when the communication weight is of zero lower bound. The conditional flocking emerges for the case of constant noise intensity, while the unconditional flocking emerges for various time-varying intensities and long-range communications.
03.12.2020 | 14:30 — 15:30 Europe/Lisbon
Youcef Mammeri (Université de Picardie Jules Verne)
A SIR-type model with diffusion to describe the spatial spread of Covid-19
We all have to deal with the coronavirus epidemic. Many strategies have been put in place to try to contain the disease, with varying success.
I will present an SIR-type mathematical model to predict the state of the epidemic. The effect of distancing, isolation of exposed individuals and treatment of symptoms will be compared.
I will begin with a simple explanation of SIR models, then discuss a PDE model and its resolution.
26.11.2020 | 13:30 — 14:30 Europe/Lisbon
Jean-Francois Babadjian (Université Paris Saclay)
Concentration versus oscillation effects in brittle damage
This talk is concerned with the asymptotic analysis of a variational model of brittle damage, when the damaged zone concentrates into a set of zero Lebesgue measure, and, at the same time, the stiffness of the damaged material becomes arbitrarily small. In a particular non-trivial regime, concentration leads to a limit energy with linear growth as typically encountered in perfect plasticity. While the singular part of the limit energy can be easily described, the identification of the bulk part of the limit energy requires a subtler analysis of the interplay between concentration and oscillation properties of the displacements.
This is a joint work with F. Iurlano and F. Rindler.
26.11.2020 | 14:30 — 15:30 Europe/Lisbon
Marco Caroccia (Università degli studi di Roma Tor Vergata)
Contact surface of Cheeger sets
Geometrical properties of Cheeger sets have been deeply studied by many authors since their introduction, as a way of bounding from below the first Dirichlet (p)-Laplacian eigenvualue. They represents the first eigenfunction of the Dirichlet (1)-Laplacian of a domain. In this talk we will introduce a recent property, studied in collaboration with Simone Ciani (Università degli studi di Firenze), concerning their contact surface with the ambient space. In particular we will show that the contact surface cannot be too small, with a lower bound on the dimension strictly related to the regularity of the ambient space. The talk will focus on the introduction of the problem. It will start with a brief explanation of its connection with the Dirichlet (p)-Laplacian eigenvalue problem. Then a brief sketch of the proof is given. Functional to the whole argument is the notion of removable singularity, as a tool for extending solutions of pdes under some regularity constraint.
26.11.2020 | 13:30 — 14:30 Europe/Lisbon
Jean-Francois Babadjian (Université Paris Saclay)
Concentration versus oscillation effects in brittle damage
This talk is concerned with the asymptotic analysis of a variational model of brittle damage, when the damaged zone concentrates into a set of zero Lebesgue measure, and, at the same time, the stiffness of the damaged material becomes arbitrarily small. In a particular non-trivial regime, concentration leads to a limit energy with linear growth as typically encountered in perfect plasticity. While the singular part of the limit energy can be easily described, the identification of the bulk part of the limit energy requires a subtler analysis of the interplay between concentration and oscillation properties of the displacements.
This is a joint work with F. Iurlano and F. Rindler.
26.11.2020 | 14:30 — 15:30 Europe/Lisbon
Marco Caroccia (Università degli studi di Roma Tor Vergata)
Contact surface of Cheeger sets
Geometrical properties of Cheeger sets have been deeply studied by many authors since their introduction, as a way of bounding from below the first Dirichlet (p)-Laplacian eigenvualue. They represents the first eigenfunction of the Dirichlet (1)-Laplacian of a domain. In this talk we will introduce a recent property, studied in collaboration with Simone Ciani (Università degli studi di Firenze), concerning their contact surface with the ambient space. In particular we will show that the contact surface cannot be too small, with a lower bound on the dimension strictly related to the regularity of the ambient space. The talk will focus on the introduction of the problem. It will start with a brief explanation of its connection with the Dirichlet (p)-Laplacian eigenvalue problem. Then a brief sketch of the proof is given. Functional to the whole argument is the notion of removable singularity, as a tool for extending solutions of pdes under some regularity constraint.
16.11.2020 | 14:30 — 15:30 Europe/Lisbon
Hynek Kovařík (Università degli studi di Brescia)
Absence of positive eigenvalues of magnetic Schroedinger operators
We study sufficient conditions for the absence of positive eigenvalues of magnetic Schroedinger operators in . In our main result we prove the absence of eigenvalues above certain threshold energy which depends explicitly on the magnetic and electric field. A comparison with the examples of Miller-Simon shows that our result is sharp as far as the decay of the magnetic field is concerned.
The talk is based on a joint work with Silvana Avramska-Lukarska and Dirk Hundertmark.
**Please note that this seminar will take place Monday, not the usual day of the Lisbon WADE.**
12.11.2020 | 14:30 — 15:30 Europe/Lisbon
Rainer Mandel (Karlsruhe Institute of Technology)
New dual variational methods for Nonlinear Helmholtz Equations and polychromatic solutions of Nonlinear wave equations
In the first part of my talk, I present the classical dual variational method in the context of Nonlinear Helmholtz equations that describe monochromatic waves in nonlinear materials. Afterwards, I discuss two recent generalizations of the method. The first deals with an extension to Nonlinear Helmholtz equations with sign-changing nonlinearities. For these problems we construct solutions that have infinite Morse-Index in the dual variational formulation. The second generalization concerns dual variational methods for the construction of breathers, i.e., polychromatic, spatially localized and time-periodic solutions of nonlinear wave equations.
The results were obtained in collaboration with D. Scheider and T. Yesil.
5/11/2020, 14:30 — 15:30 Europe/Lisbon
Wladimir Neves (Universidade Federal do Rio de Janeiro)
Homogenization of Schrödinger equations. Extended Effective Mass Theorems for non-crystalline matter.
In this talk, we study the homogenization of the Schrödinger equation beyond the periodic setting. More precisely, rigorous derivation of the effective mass theorems in solid state physics for non-crystalline materials are obtained.
This is a joint work with Vernny Ccajma and Jean Silva.
29/10/2020, 13:30 — 14:30 Europe/Lisbon
Lorenzo Mazzieri and Piotr Chruściel (Università di Trento / University of Vienna)
Static vacuum black holes and Lambda
We will review the status of the uniqueness theory of static vacuum black holes, with or without a cosmological constant and we will outline the proof of a uniqueness theorem with
, proved jointly in collaboration with Stefano Borghini.
22/10/2020, 14:30 — 15:30 Europe/Lisbon
Jean-Baptiste Casteras (CMAFcIO, Universidade de Lisboa)
Standing wave and travelling wave solutions for a fourth order Schrödinger equation
In this talk, we will be interested in standing wave solutions to a fourth order nonlinear Schrödinger equation having second and fourth order dispersion terms. This kind of equation naturally appears in nonlinear optics. In a first time, we will establish the existence of ground-state and renormalized solutions. We will then be interested in their qualitative properties, in particular their stability.
Joint works with Denis Bonheure, Ederson Moreira Dos Santos, Tianxiang Gou, Louis Jeanjean and Robson Nascimento.
04/09/2020, 11:00–12:00 Europe/Lisbon — Online *
Marco Morandotti (Politecnico di Torino)
Spatially inhomogeneous evolutionary games
We study an interaction model of a large population of players based on an evolutionary game, which describes the dynamical process of how the distribution of strategies changes in time according to their individual success.
We assume that the population of players is distributed over a state space and that they are each endowed with probability distributions of pure strategies, which they draw at random to evolve their states. Simultaneously, the mixed strategies evolve according to a replicator dynamics, modeling the success of pure strategies according to a payoff functional.
We establish existence, uniqueness, and stability of Lagrangian and Eulerian solutions of this dynamical game by using methods of ODE and optimal transport on Banach spaces.
We apply the general theoretical framework to perform the mean-field analysis of a multi-population agent-based model, where a particle dynamics derived by a nonlocal velocity and a Markov-type jump process for the label s are coupled.
An alternate Lagrangian approximation scheme at discrete times is also proposed.
This research is from joint works with S. Almi, L. Ambrosio, M. Fornasier, G. Savaré, and F. Solombrino.
14/07/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Pavel Exner (Doppler Institute for Mathematical Physics and Applied Mathematics, Prague)
Vertex coupling and spectra of periodic quantum graphs.
The talk focuses on the influence of the vertex coupling on spectral properties of periodic quantum graphs. Specifically, two questions will be addressed. The first concerns the number of open spectral gaps; it is shown that graphs with a nontrivial coupling can have finite but nonzero number of them. Secondly, motivated by recent attempts to model the anomalous Hall effect, we investigate a class of vertex couplings that violate the time reversal invariance. For the simplest coupling of this type we show that its high-energy properties depend on the parity of the lattice vertices, and discuss various consequences of this property.
*to get access to the password, please register on the website or contact one of the organizers.
09/07/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Elvira Zappale (Università Degli Studi di Salerno)
Optimal design problemsI will present several integral representation results for certain functionals arising in the context of optimal design and damage models, in presence of a perimeter penalization term.
I will consider several frameworks, and I will also discuss the case with non-standard growth conditions.
*to get access to the password, please register on the website or contact one of the organizers.
30/06/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Barbara Brandolini (Università Degli Studi di Napoli Federico II)
Sharp lower bounds for Neumann eigenvalues
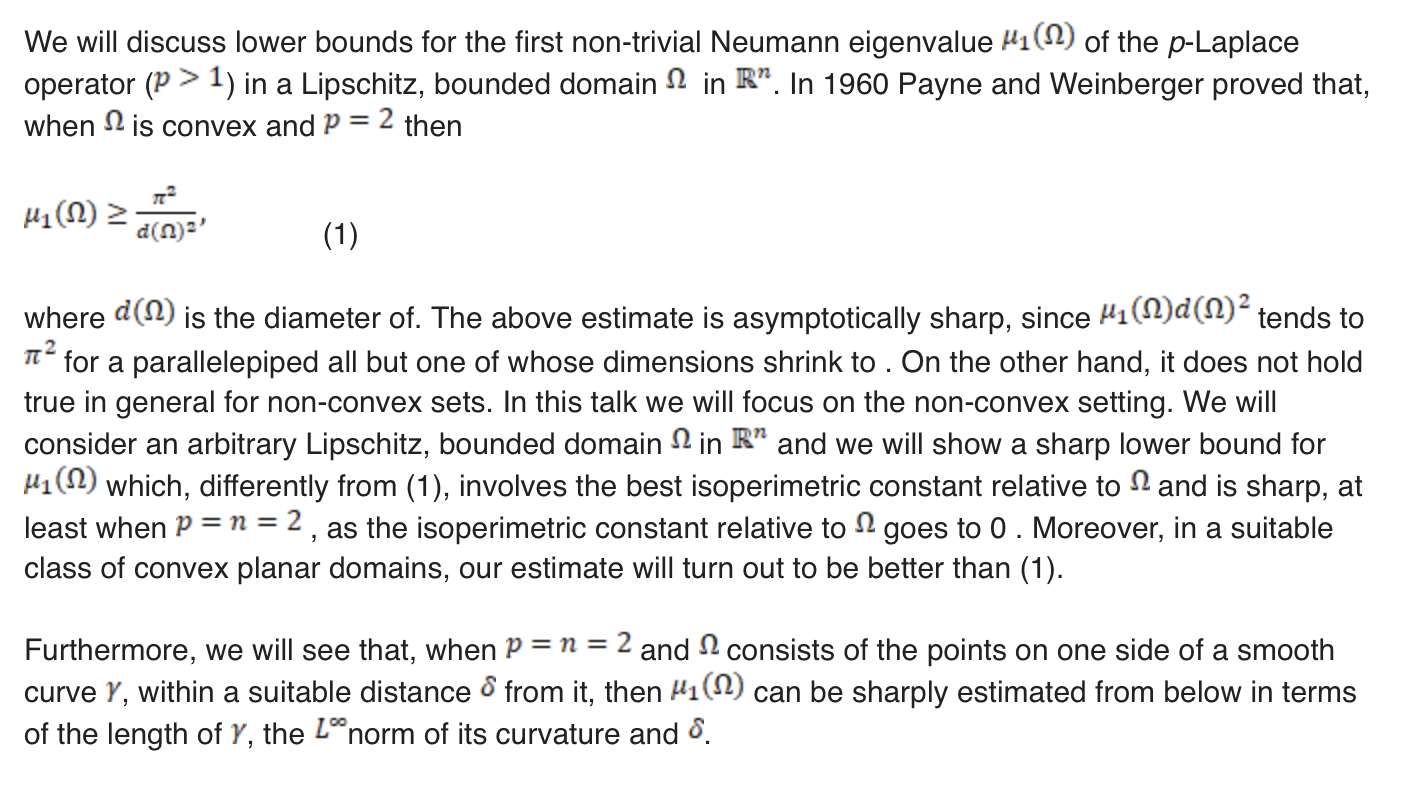
*to get access to the password, please register on the website or contact one of the organizers.
23/06/2020, Tuesday, 11:00–12:00 Europe/Lisbon — Online *
Tatsuya Miura (Tokyo Institute of Technology)
On the isoperimetric inequality and surface diffusion flow for multiply winding curves.
In this talk we discuss dynamical stability of multiply covered circles under the surface diffusion flow. To this end we first establish a general form of the isoperimetric inequality for immersed closed curves under rotational symmetry, which would be of independent interest. We then apply it to obtaining a certain class of rotationally symmetric initial curves from which solutions to the surface diffusion flow exist globally-in-time and converge to multiply covered circles. This talk is based on a joint work with Shinya Okabe at Tohoku University.
*to get access to the password, please register on the website or contact one of the organizers.
16/06/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Riccardo Adami (Politecnico di Torino)
Ground states of the Nonlinear Schroedinger Equation on Graphs: an overview.
Driven by physical and technological applications, during the last five years the study of nonlinear evolution on branched structures (graphs, networks) has undergone a fast development. We review on the main achievements and on the open problems. This is a joint project with several people, among which Simone Dovetta, Enrico Serra, Lorenzo Tentarelli, and Paolo Tilli.
*to get access to the password, please register on the website or contact one of the organizers.
09/06/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Lucio Boccardo (Università di Roma La Sapienza)
Recent developments on Dirichlet problems with singular convection/drift terms.

*to get access to the password, please register on the website or contact one of the organizers.
02/06/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Maria Colombo (École Polytechnique Fédérale de Lausanne)
Nonunique characteristic curves of Sobolev vector fields
Given a vector field in , the classical Cauchy-Lipschitz theorem shows existence and uniqueness of its flow provided the vector field is sufficiently smooth; this, in turn, translates in existence and uniqueness results for the transport equation. In 1989, Di Perna and Lions proved that Sobolev regularity for vector fields, with bounded divergence and a growth assumption, is sufficient to establish existence, uniqueness and stability of a generalized notion of flow, consisting of a suitable selection among the trajectories of the associated ODE. A long-standing open question is whether the uniqueness of the regular Lagrangian flow is a corollaryof the uniqueness of the trajectory of the ODE for a.e. initial datum. In this talk we give an overview of the topic and we provide a negative answer to this question. To show this result we exploit the connection with the transport equation, based on Ambrosio’s superposition principle, and a new ill-posedness result for positive solutions of the continuity equation.
*to get access to the password, please register on the website or contact one of the organizers.
26/05/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Luis Vega (Basque Center for Applied Mathematics)
The Vortex Filament Equation, the Talbot effect, and non-circular jets
We will propose the vortex filament equation as a possible toy model for turbulence, in particular because of its striking similarity to the dynamics of non-circular jets. This similarity implies the existence of some type of Talbot effect due to the interaction of non-linear waves that propagate along the filament. Another consequence of this interaction is the existence of a new class of multi-fractal sets that can be seen as a generalization of the graph of Riemann’s non-differentiable function. Theoretical and numerical arguments about the transfer of energy will be also given. This a joint work with V. Banica and F. de la Hoz.
*to get access to the password, please register on the website or contact one of the organizers.
19/05/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Bernold Fiedler (Institute of Mathematics, Freie Universität Berlin)
Sturm meanders: global attractors, Temperley-Lieb algebras, and black holes
Fusco and Rocha studied Neumann boundary value problems for scalar ODEs of second order via a shooting approach. They introduced the notion of what we now call Sturm permutations. These permutations relate, on the one hand, to a special class of meandering curves as introduced by Arnol’d in a singularity theory context. On the other hand, they became central in the study of global attractors of nonlinear parabolic partial differential equations of Sturm type.
We discuss relations of Sturm meanders with further areas: the multiplicative and trace structure in Temperley-Lieb algebras, discrete versions of Cartesian billiards, and the problem of constructing initial conditions for black hole dynamics which satisfy the Einstein constraints. We also risk a brief glimpse at the long and meandric history of meander patterns themselves.
We report on joint work with Pablo Castañeda, Juliette Hell, Carlos Rocha, and Brian Smith. See also http://dynamics.mi.fu-berlin.de/
For further material we recommend the beautifully illustrated book “Meanders” by Anna Karnauhova, de Gruyter 2017.
*to get access to the password, please register on the website or contact one of the organizers.
12/05/2020, Tuesday, 16:30–17:30 Europe/Lisbon — Online *
Diogo Oliveira e Silva (University of Birmingham)
Global maximizers for spherical restriction
*to get access to the password, please register on the website or contact one of the organizers.
05/05/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online *
Filippo Santambrogio (Université Claude Bernard - Lyon 1)
Optimal transport methods for the regularity of 2D functions of least gradient
The least gradient problem (minimizing the BV norm with given boundary data), motivated by both image processing applications and connections with minimal surfaces, is known to be equivalent, in the plane, to the Beckmann minimal-flow problem (an alternative formulation of the L1 Monge-Kantorovich optimal transport problem) with source and target measures located on the boundary of the domain. Hence, Sobolev regularity of functions of least gradient is equivalent in this setting to Lp bounds on the solution of the Beckmann problem (i.e. on what is called the transport density) and can be attacked with techniques which are now standard in optimal transport. From the transport point of view, the novelty of the estimates that I will present, coming from a joint paper with S. Dweik, lies in the fact they are obtained for transport between measures which are concentrated on the boundary. From the BV point of view, a new result is the W1,p regularity of the least gradient function whenever the boundary datum is W1,p as a 1D function: moreover, the optimal transport framework is strong enough to deal with arbitrary strictly convex norms instead of the Euclidean one with almost no effort.
*to get access to the password, please register on the website or contact one of the organizers.
The Lisbon Webinar in Analysis in Differential Equations is a joint iniciative of CAMGSD, CMAFcIO and GFM, three research centers of the University of Lisbon. It is aimed at filling the absence of face-to-face seminars and wishes to be a meeting point of mathematicians working in the field.
28/04/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online
Michael Goldman (Laboratoire Jacques-Louis Lions and Université Paris 7)
On an old conjecture of Almgren
In this talk I will give an overview on the few results available on the conjecture of Almgren regarding the convexity of drops subject to the action of an external potential. In particular I will present recent progress in this direction obtained with G. De Philippis on their connectedness. Together with an older result of McCann, this answers positively the conjecture in dimension two. The proof is inspired by the two-point function technique introduced by B. Andrews and is reminiscent of the doubling of variables trick in the context of viscosity solutions.
21/04/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online
Matthias Röger, Technische Universität Dortmund
An obstacle type problem as a limit of a model for cell polarization
We consider the polarization of a cell in response to an outer signal. The mathematical model consists of a diffusion equation in the inner volume coupled to a reaction diffusion system on the cell membrane. In a certain asymptotic limit we rigorously prove the convergence towards a generalized obstacle problem. In term of this limit system we derive conditions for the onset of polarization. The results will be mainly presented for the stationary case, we will briefly discuss some extensions to the time-dependent case.
(This is joint work with Barbara Niethammer, Juan Velazquez, and Anna Logioti)
14/04/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online
Dorin Bucur, Université de Savoie.
Boundary behaviour of Robin problems and isoperimetric spectral inequalities.
Consider the Poisson equation with Robin boundary conditions in a (nonsmooth) domain with a bounded, nonnegative right hand side. Given a point on the boundary, the question is whether the solution has a strictly positive lower limit at this point. If the domain is smooth the answer is positive as a consequence of the Hopf maximum principle. If the domain is not smooth, the answer may be positive or negative, depending on the geometry of the domain around the point. This question was raised in a probabilistic context by Bass, Burdzy and Chen in 2008, when they obtained results for Lipschitz sets and cuspidal domains.
Our motivation is related to the fact that positive answers to the question above, together with a control of the infimum of the boundary values, lead to sharp quantitative forms of isoperimetric inequalities of spectral type for the Robin Laplacian.
In this talk, I will make the point on recent advances on isoperimetric inequalities involving the Robin Laplacian and I will show how the boundary behaviour pops up in the quantitative form of the inequalities. If times remains, I will present a variational approach to deal with the boundary behaviour for general elliptic operators and various geometric contexts.
The results were obtained together with A. Giacomini and M. Nahon.
07/04/2020, Tuesday, 16:00–17:00 Europe/Lisbon — Online
Susanna Terracini, Università di Torino.
Pattern Formation Through Spatial Segregation.

Video available here.
